We cannot live without energy.

- Chemical energy in food we eat is converted by or body to mechanical energy to move our hands and legs. But not all of it is converted. There is still chemical energy in what we pass out after our food is digested!

- The chemical energy in animals and plants we eat comes from photosynthesis using light energy from the sun. Likewise, not all the sunlight falling on a leaf is converted into chemical energy.

- Sunlight is produced by nuclear reaction of hydrogen in the sun (that is to say - sunlight is nuclear energy!) And of course, most of sun's energy are lost in outer space.

When we spend long hours doing our best at our studies or our job, we are doing a lot of work. When we work for long hours without sleeping, we have a lot of energy. When can lift heavy objects, we have a lot of power.

Work, energy and power are words that are often used in everyday life. We may choose one word or another just to make the sentence more interesting or emphatic, depending on our mood.

Because these three words - work, energy and power - are so familiar, many students get very confused when they study this topic in physics.

The problem is that in physics, these words have meanings that are quite different - yet not completely different - from the way we normally use them. Each has a specific meaning that is different from the other two, so we cannot just use whichever word we like based on our mood.

It is almost like a set of new meanings - different yet not completely different. That makes it a sure recipe for confusion.

What is work, really?

So if work is not force, energy nor power in physics, then what is it?

As simple example - lets say I push a box on the floor with a certain force over a straight distance. Then I have done some work. The work that I have done on the box is equal to the value of the force I pushed, multiplied by the distance the tbe box is moved.

So work done on the box is force times distance !

But what about my schoolwork and my homework? That is where we have to remind ourselves that "work" in physics do not have the same meaning as work in schoolwork and homework, even if we may get even more tired doing them.

With this starting point - a special and very specific meaning for "work", further ideas and methods are then developed.

Lets say I am compressing a gas. I cannot actually push a gas or press a gas with my bare hands, as a gas is not a solid object. But in physics, people often find ways to get round small problems like this. They call it experiments.

For example - we can use a balloon or a bicycle pump. When I press on a bicycle pump connected to a bicycle tyre, I apply a force and push the pump handle over a distance. That is work done !

As it turns out, there is another way to calculate this work apart from force times distance. It is pressure times the change in volume of the air when we press it into the tyre!

If you think the physics meaning of work is troublesome, wait till you hear the physics definition of energy:

Energy is the capacity to do work.

??!!

If that is how you feel when you see this, I am equally mystified even after nearly half a century studying and working in physics.

Students eventually learn how to do calculations on "energy" correctly, engineers have obviously build fantastic devices that run our modern world using all sorts of "energy".

In today's world and news, we hear a lot about energy. Fossil fuel like petrol and gas came from maybe dead plants and animals buried underground for millions of years. The energy in fossil fuel originally came from the sun through photosynthesis. The sun got its energy from nuclear fusion of hydrogen atoms in the sun ...

Sounds complicated ? I think so too.

So we kind of know energy is important, and that we need it to do all the things in life that we want to do. Including to study physics !

Ok, back to physics. So the definition of energy in physics lesson does not seem very clear. It is actually the physics exercises and problems we do that kind of clarify the idea of energy.

A few examples :

- we learn that energy is the capacity (ability?) to do work
- we learn to calculate work done
- we learn formulae to calculate different types of energy (light, heat, mechanical, electrical)
- we learn the energy can be converted from one form to another (e.g. light to electricity using solar panels)

With some patience and practice, a student would know this like the back of his or her hand ...

The are many devices around us that helps us with our life. These devices converts energy from a convenient form that we can buy, to a form that we need. For example :

- the fan converts electrical energy to mechanical energy of wind movement

- the candle converts chemical energy of wax to light energy

- solar panel converts light energy into electrical energy

These very different methods have one thing in common - none of them can convert 100% percent of one type of energy to the other.

No matter how well a fan is made, it cannot convert 100% of electrical energy to wind energy. There would be friction in the rotating motor, and resistance in the electrical wire that wastes some of the energy.

The candle burning would of course produce a lot of heat that we may not want - if we just need the light.

Solar panel - efficiency is 15% to 20%. This means that only 15% to 20% of the sunlight falling on a solar panel is converted to electricity. The rest probably get reflected off or warms up the panel.

It is like saying nothing is perfect. This seems almost like a law of nature - 100% efficiency just does not seem to be possible.

I have so far written about work and energy in a decriptive way. The closest I have come to mathematics is the mentioning above that

work done = force x distance

Without going into the details, I shall just mention here that using this equation and those from kinematics, it is possible to work through the mathematics and relate this to the velocity of the mass that is pushed by that force :

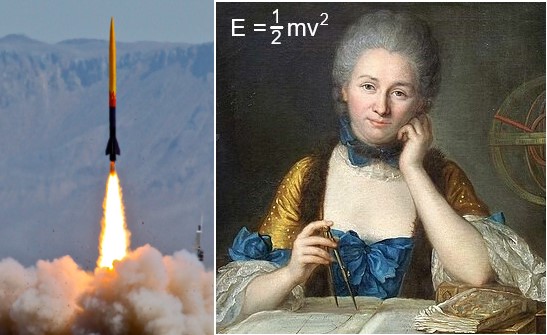
kinetic energy = 1/2 x mass x velocity squared.

This correct formula was derived by a French lady Émilie du Châtelet in the 18th century. Newton tried but missed out the 1/2. Students may do that sometimes too.

What really amazes me is that the velocity, work and energy, three things in nature that seem very different, can be related exactly by such simple mathematics.

But apart from people who like physics, and apart from giving students a hard time in exams, what is the use of making students learn this kinetic energy formula?

Actually, it can make doing exam questions easier. Some questions that are difficult to solve by calculating forces - may be easier to do using kinetic energy. If that is hard to believe, here is an example:

If you drop a ball from a 1 m height, with what speed does it hit the ground?

You can use the acceleration equations which are more complicated. Or you can just say the potential energy at 1 m height is all changed to kinetic energy when it hits the ground.

So kinetic energy = potential energy

Then with some simple algebra, you can use this to calculate the speed. That's it!

The ideas and methods of potential energy are also used in other areas of phyisics - electricity and elastic material.

The effects of electricity comes from something called electrical charges. Just as object around us have mass and weight, so they also have electrical charges. There are 2 types - positive charge and negative charge.

These electric charges live in every atom. Positive charges in an atom live in the centre of the atom in tiny particles called protons. Negative charges live in electrons, which are even tinier particles that live near and around the centre.

Positive end negative electric charges attract each other. As a result, their energies and movements can also be calculated using techniques above on potential energy and kinetic energy.

Springs and elestic materials can also have potential energy. When we stretch a rubber band, we do work and our energy iis stored in the rubber band as potential energy. When we let go, the rubber band springs back - so the elastic potential energy is converted back to kinetic energy.

In another of my other blog - on the topic of kinematics in the physics syllabus

- I have mentioned that if we plot of graph of the velocity of a moving object against time, the the area under that graph is actually equal to the distance the the object moves.

This is quite useful in physics calculations. The graph of force on an object - plotted against the distance the object moves - has a similar property. The area under the graph is the work done on the object !

Suppose we pull slowly on a spring and plot a graph of the force on the spring against its extension. Then the work done on the spring is equal to the are under the graph!

The above connection means that the reverse is also true. Just as we can find the acceleration using the gradient of a velocity time graph, so we can find the force from the gradient of a potential energy versus distance graph.

If you have been patient enough to read this far, the congratulations. You have "potential energy" to do well in physics !

You can learn these concepts and more at Dr Hock's maths and physics tuition.